Currently Empty: ₹0.00
Cuisenaire Strip
₹478.00
Cuisenaire Strip
AGE GROUP:
- Suitable for children of above 6 to ……………years
- Recommended for grade 1,2,3,4,5,6 and 7
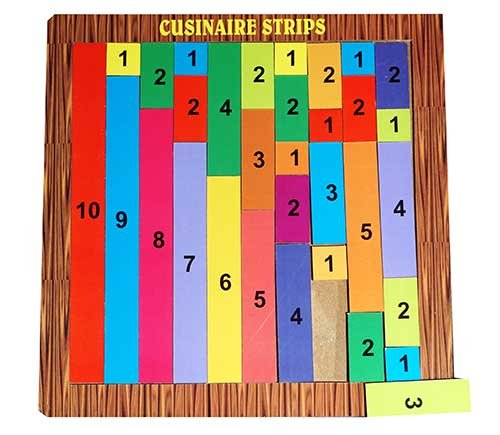
INSIDE THE BOX:
- Rectangular board with a cavity to hold strips called rods.
- Each rod is of distinct colours and number.
- Activity sheet
LEARNING OUTCOME:
- Playing with the rods helps in understanding the concept of whole number, fraction, ration and proportion, LCM, HCF etc.
- While playing children discover corelation between putting together – addition – repeated addition – multiplication. And taking away- subtraction- repeated subtraction – division
Cuisenaire Strip
CHALLENGES IN LEARNING MATHEMATICAL CONCETS:
Each mathematical concept has their independent logic and are also linked to other concept. For example, to understand basic operation, students need to understand the corelation between putting together – addition – repeated addition – multiplication. Students often lack this. Sometimes they also faulter in understanding the independent logic of each concept. Case in this point is the concept of whole and fractional number. Often student’s extent the logic of whole numbers to understand fractional number. For example, if we ask students to compare fraction,4/6 and 3/2 . Most of students would consider 4/6 > 3/2. This is because 4 and 6 are greater whole numbers then 3 and 2.
WHY CUISENAIIRE STRIP?
Rods representing numbers are put together to understand basic operation. Children understand that 2 rods and 3 rods make 5 rods. If we add 5 rods 4 times, it makes 20 rods. Gradually students understand the corelation between putting together – addition – repeated addition – multiplication.
Difference in logic of whole and fractional number becomes clear when students start constructing fractional numbers using Cuisenaire strips. They understand that to compare two unlike fractions, first we need to convert the unlike fraction 4/6 and 3/2 into like fraction 4/6 and 9/6 where denominator is same. Now if we compare, we realise that second number is greater than first number. It seems so easy because visualisation is possible with the concrete rods of Cuisenaire strip.